Radiocarbon Dating
- Page ID
- 1479
- Identify the age of materials that can be approximately determined using Radiocarbon dating.
When we speak of the element Carbon, we most often refer to the most naturally abundant stable isotope 12C. Although 12C is definitely essential to life, its unstable sister isotope 14C has become of extreme importance to the science world. Radiocarbon dating is the process of determining the age of a sample by examining the amount of 14C remaining against its known half-life, 5,730 years. The reason this process works is because when organisms are alive, they are constantly replenishing their 14C supply through respiration, providing them with a constant amount of the isotope. However, when an organism ceases to exist, it no longer takes in carbon from its environment and the unstable 14C isotope begins to decay. From this science, we are able to approximate the date at which the organism lived on Earth. Radiocarbon dating is used in many fields to learn information about the past conditions of organisms and the environments present on Earth.
The Carbon-14 Cycle
Radiocarbon dating (usually referred to simply as carbon-14 dating) is a radiometric dating method. It uses the naturally occurring radioisotope carbon-14 (14C) to estimate the age of carbon-bearing materials up to about 58,000 to 62,000 years old. Carbon has two stable, nonradioactive isotopes: carbon-12 (12C) and carbon-13 (13C). There are also trace amounts of the unstable radioisotope carbon-14 (14C) on Earth. Carbon-14 has a relatively short half-life of 5,730 years, meaning that the fraction of carbon-14 in a sample is halved over the course of 5,730 years due to radioactive decay to nitrogen-14. The carbon-14 isotope would vanish from Earth's atmosphere in less than a million years were it not for the constant influx of cosmic rays interacting with molecules of nitrogen (N2) and single nitrogen atoms (N) in the stratosphere. Both processes of formation and decay of carbon-14 are shown in Figure 1.
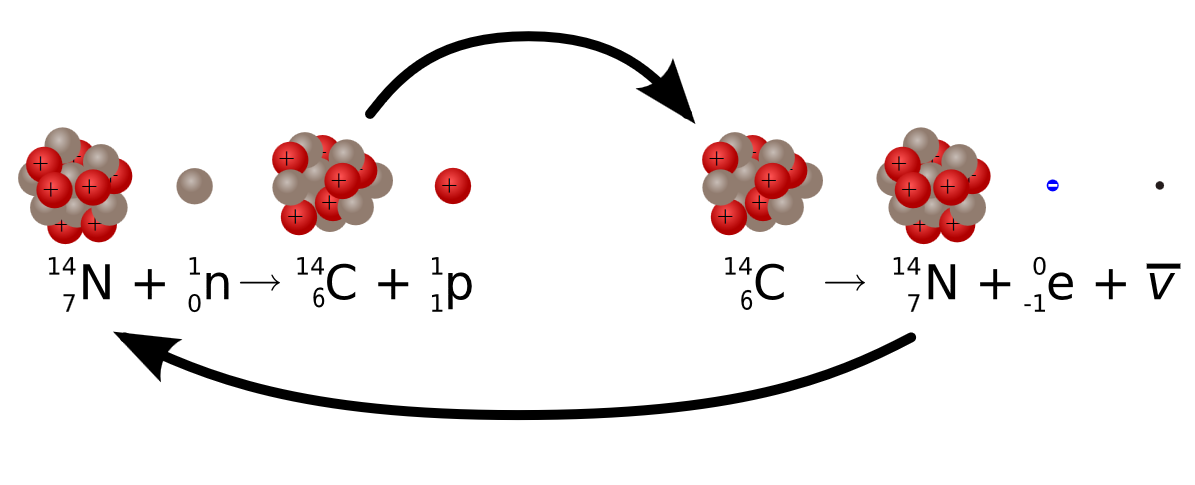
When plants fix atmospheric carbon dioxide (CO2) into organic compounds during photosynthesis, the resulting fraction of the isotope 14C in the plant tissue will match the fraction of the isotope in the atmosphere (and biosphere since they are coupled). After a plant dies, the incorporation of all carbon isotopes, including 14C, stops and the concentration of 14C declines due to the radioactive decay of 14C following.
\[ \ce{ ^{14}C -> ^{14}N + e^-} + \mu_e \label{E2}\]
This follows first-order kinetics:
\[N_t= N_o e^{-kt} \label{E3}\]
where
- \(N_0\) is the number of atoms of the isotope in the original sample (at time t = 0, when the organism from which the sample is derived was de-coupled from the biosphere).
- \(N_t\) is the number of atoms left after time \(t\).
- \(k\) is the rate constant for the radioactive decay.
The half-life of a radioactive isotope (usually denoted by \(t_{1/2}\)) is a more familiar concept than \(k\) for radioactivity, so although Equation \(\ref{E3}\) is expressed in terms of \(k\), it is more usual to quote the value of \(t_{1/2}\). The currently accepted value for the half-life of 14C is 5,730 years. This means that after 5,730 years, only half of the initial 14C will remain; a quarter will remain after 11,460 years; an eighth after 17,190 years; and so on.
The equation relating rate constant to half-life for first order kinetics is
\[ k = \dfrac{\ln 2}{ t_{1/2} } \label{E4}\]
so the rate constant is then
\[ k = \dfrac{\ln 2}{5.73 \times 10^3} = 1.21 \times 10^{-4} \text{year}^{-1} \label{E5}\]
and Equation \(\ref{E2}\) can be rewritten as
\[N_t= N_o e^{-\ln 2 \;t/t_{1/2}} \label{E6}\]
or
\[t = \left(\dfrac{\ln \dfrac{N_o}{N_t}}{\ln 2} \right) t_{1/2} = 8267 \ln \dfrac{N_o}{N_t} = 19035 \log_{10} \dfrac{N_o}{N_t} \;\;\; (\text{in years}) \label{E7}\]
The sample is assumed to have originally had the same 14C/12C ratio as the ratio in the atmosphere, and since the size of the sample is known, the total number of atoms in the sample can be calculated, yielding \(N_0\), the number of 14C atoms in the original sample. Measurement of N, the number of 14C atoms currently in the sample, allows the calculation of \(t\), the age of the sample, using the Equation \(\ref{E7}\).
Deriving Equation \(\ref{E7}\) assumes that the level of 14C in the atmosphere has remained constant over time. However, the level of 14C in the atmosphere has varied significantly, so time estimated by Equation \(\ref{E7}\) must be corrected by using data from other sources.
In 1947, samples of the Dead Sea Scrolls were analyzed by carbon dating. It was found that the carbon-14 present had an activity (rate of decay) of d/min.g (where d = disintegration). In contrast, living material exhibit an activity of 14 d/min.g. Thus, using Equation \(\ref{E3}\),
\[\ln \dfrac{14}{11} = (1.21 \times 10^{-4}) t \nonumber\]
Thus,
\[t= \dfrac{\ln 1.272}{1.21 \times 10^{-4}} = 2 \times 10^3 \text{years} \nonumber\]
From the measurement performed in 1947, the Dead Sea Scrolls were determined to be 2000 years old, giving them a date of 53 BC, and confirming their authenticity. This discovery is in contrast to the carbon dating results for the Turin Shroud that was supposed to have wrapped Jesus’ body. Carbon dating has shown that the cloth was made between 1260 and 1390 AD. Thus, the Turin Shroud was made over a thousand years after the death of Jesus.
Describes radioactive half-life and how to do some simple calculations using half-life.
History
The technique of radiocarbon dating was developed by Willard Libby and his colleagues at the University of Chicago in 1949. Emilio Segrè asserted in his autobiography that Enrico Fermi suggested the concept to Libby at a seminar in Chicago that year. Libby estimated that the steady-state radioactivity concentration of exchangeable carbon-14 would be about 14 disintegrations per minute (dpm) per gram. In 1960, Libby was awarded the Nobel Prize in chemistry for this work. He demonstrated the accuracy of radiocarbon dating by accurately estimating the age of wood from a series of samples for which the age was known, including an ancient Egyptian royal barge dating from 1850 BCE. Before Radiocarbon dating was discovered, someone had to find the existence of the 14C isotope. In 1940, Martin Kamen and Sam Ruben at the University of California, Berkeley Radiation Laboratory did just that. They found a form, an isotope, of Carbon that contained 8 neutrons and 6 protons. Using this finding, Willard Libby and his team at the University of Chicago proposed that Carbon-14 was unstable and underwent a total of 14 disintegrations per minute per gram. Using this hypothesis, the initial half-life he determined was 5568, give or take 30 years. The accuracy of this proposal was proven by dating a piece of wood from an Ancient Egyptian barge, the age of which was already known. From that point on, scientists have used these techniques to examine fossils, rocks, and ocean currents; as well as to determine age and event timing. Throughout the years, measurement tools have become more technologically advanced, allowing researchers to be more precise. We now use what is known as the Cambridge half-life of 5730+/- 40 years for Carbon-14. Although it may be seen as outdated, many labs still use Libby's half-life in order to stay consistent in publications and calculations within the laboratory. From the discovery of Carbon-14 to radiocarbon dating of fossils, we can see what an essential role Carbon has played and continues to play in our lives today.
Summary
The entire process of Radiocarbon dating depends on the decay of carbon-14. This process begins when an organism is no longer able to exchange Carbon with its environment. Carbon-14 is first formed when cosmic rays in the atmosphere allow for excess neutrons to be produced, which then react with Nitrogen to produce a constantly replenishing supply of carbon-14 to exchange with organisms.
- Carbon-14 dating can be used to estimate the age of carbon-bearing materials up to about 58,000 to 62,000 years old.
- The carbon-14 isotope would vanish from Earth's atmosphere in less than a million years were it not for the constant influx of cosmic rays interacting with atmospheric nitrogen.
- One of the most frequent uses of radiocarbon dating is to estimate the age of organic remains from archeological sites.
References
- Hua, Quan. "Radiocarbon: A Chronological Tool for the Recent Past." Quaternary Geochronology 4.5(2009):378-390. Science Direct. Web. 22 Nov. 2009.
- Petrucci, Ralph H. General Chemistry: Principles and Modern Applications 9th Ed. New Jersey: Pearson Education Inc. 2007.
- "Radio Carbon Dating." BBC- Homepage. 25 Oct. 2001. Web. 22 Nov. 2009. http://www.bbc.co.uk.
- Willis, E.H., H. Tauber, and K. O. Munnich. "Variations in the Atmospheric Radiocarbon Concentration Over the Past 1300 Years." American Journal of Science Radiocarbon Supplement 2(1960) 1-4. Print.
Problems
- If, when a hippopotamus lived, there was a total of 25 grams of Carbon-14, how many grams will remain 5730 years after he is laid to rest? 12.5 grams, because one half-life has occurred.
- How many grams of Carbon-14 will be present in the hippopotamus' remains after three half-lives have passed? 3.125 grams of Carbon-14 will remain after three half-lives.
Contributors and Attributions
- Template:ContribBarron
Boundless (www.boundless.com)
- Wikipedia

