Electronic Angular Wavefunction
- Page ID
- 2524
The electronic angular wavefunction is one spatial component of the electronic Schrödinger wave equation, which describes the motion of an electron. It depends on angular variables, \(\theta\) and \(\phi\), and describes the direction of the orbital that the electron may occupy. Some of its solutions are equal in energy and are therefore called degenerate.
Introduction
Electrons can be described as a particle or a wave. Because they exhibit wave behavior, there is a wavefunction that is a solution to the Schrödinger wave equation:
\[\hat{H}\Psi(r,\phi,\theta,t)=E\Psi(r,\phi,\theta,t)\]
This equation has eigenvalues, \(E\), which are energy values that correspond to the different wavefunctions.
Spherical Coordinates
To solve the Shrödinger equation, spherical coordinates are used. Spherical coordinates are in terms of a radius \(r\), as well as angles \(\phi\), which is measured from the positive x axis in the xy plane and may be between 0 and \(2\pi\), and \(\theta\), which is measured from the positive z axis towards the xy plane and may be between 0 and \(\pi\).
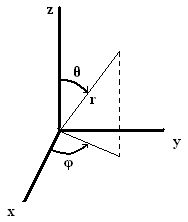
\(x=rsin(\theta)cos(\phi)\)
\(y=rsin(\theta)sin(\phi)\)
\(z=rcos(\theta)\)
Electronic Wavefunction
The electronic wavefunction, \(\Psi(r,\phi ,\theta ,t)\), describes the wave behavior of an electron. Its value is purely mathematical and has no corresponding measurable physical quantity. However, the square modulus of the wavefunction, \(\mid \Psi(r,\phi ,\theta ,t)\mid ^2\) gives the probability of locating the electron at a given set of values. To use separation of variables, the wavefunction can be expressed as
\(\Psi(r,\phi, \theta ,t)=R(r)Y_{l}^{m}(\phi, \theta)\)
\(R(r)\) is the radial wavefunction and \(Y_{l}^{m}(\phi, \theta)\) is the angular wavefunction. Separating the angular variables in \(Y_{l}^{m}(\phi, \theta)\) gives
\(Y_{l}^{m}(\phi, \theta)=\left[\dfrac{2l+1}{4\pi}\left(\dfrac{(l-\mid m \mid)!}{(l+\mid m \mid)!}\right)\right]^{\frac{1}{2}}P_l^{\mid m \mid}(cos(\theta))e^{im\phi}\)
where \(P_l^{\mid m \mid}(cos(\theta))\) is a Legendre polynomial and is only in terms of the variable \(\theta\). The exponential function, which is only in terms of \(\phi\), determines the phase of the orbital.
For the angular wavefunction, the square modulus gives the probability of finding the electron at a point in space on a ray described by \((\phi, \theta)\). The angular wavefunction describes the spherical harmonics of the electron's motion. Because orbitals are a cloud of the probability density of the electron, the square modulus of the angular wavefunction influences the direction and shape of the orbital.
Quantum Numbers and Orbitals
There are 3 quantum numbers defined by the Schrödinger wave equation. They are \(n\), \(l\), and \(m_{l}\). Each combination of these quantum numbers describe an orbital. Values for \(n\) come from from the radial wavefunction. \(n\) may be 1, 2, 3... Because they evolved from the separation of variables performed to solve the wavefunction, solutions to the angular wavefunction are quantized by the values for \(l\) and \(m_{l}\). Acceptable values for \(l\) are given by \(l=n-1\). The corresponding values for \(m_{l}\) are integers between \(-l\) and \(+l\).
Degeneracy and p, d and f Orbitals
Orbitals descrbed by the same \(n\) and \(l\) values but different \(m_{l}\) values are degenerate, meaning that they are equal in energy but vary in their direction and, sometimes, shape. For \(p\) orbitals, \(l=1\), giving three \(m_{l}\) values and thus, 3 degenerate states. They are \(p_{x}\), \(p_{y}\)and \(p_{z}\). \(d\) orbitals have \(l=2\), giving 5 degenerate states. These are \(d_{xy}\), \(d_{xz}\), \(d_{yz}\), \(d_{z^2}\), \(d_{x^2-y^2}\). \(f\) orbitals have \(l=3\), giving a total of 7 degenerate states.
References
- McMahon, David. (2006) Quantum Mechanics Demystified New York: McGraw-Hill.
- McGervey, J. D. (1995) Quantum Mechanics:concepts and applications San Diego:Academic Press.
Problems
1. Which quantum numbers depend on the angular wavefunction?
2. Give the quantum numbers defined by the angular wavefunction for a \(d_{z^2}\) orbital.
3. Given that the spherical representation of the \(d_{x^2-y^2}\) orbital is \(r^2 sin^{2}(\theta) cos(2\phi)\), show that this matches the label for the orbital, \(x^2-y^2\). Hint: \(cos(2x)=cos^2(x)-sin^2(x)\)
Solutions:
1. \(l\) and \(m_{l}\)
2. \(l=2\) and \(m_{l}=2,1,0,-1,-2\)
3. \(r^2 sin^{2}(\theta)cos(2\phi)=r^2 sin^{2}(\theta)(cos^2(\phi)-sin^2(\phi))\)
\(r^2 sin^{2}(\theta)cos^2(\phi)-r^2 sin^{2}(\theta)sin^2(\phi)\)
Since \(x=rsin(\theta)cos(\phi)\) and \(y=rsin(\theta)sin(\phi)\),
\(r^2 sin^{2}(\theta) cos(2\phi)=x^2-y^2\)
Contributors and Attributions
- Bryn Ellison

